Interferometric Optics

These are references on the optics derived from the application of Dirac's bra-ket notation to N-slit interferometry. As explained in the references Dirac's quantum approach is applicable to the propagation of a single photon or to the propagation of ensembles of indistinguishable photons.

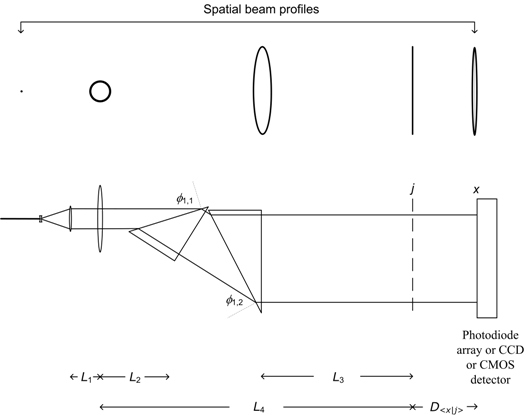
Optical architecture for the multiple-prism beam expander microscope/nanoscope (MPBEM/N). The beam incident on the object can be, for example, 25-60 mm wide X 20 µm high. This is an extremely elongated beam (in the plane of propagation) with a width to height ratio in the range of 1000:1 to 3000:1. This concept was first applied to microscopy in 1987 and to nanoscopy in 1995.

Comparisons between theory and experiments, in the NSLI configuration, have been performed for even values (N = 2, 4, 6...) and odd values (N = 3, 5, 7...) of N. This includes the cases of two-slit interference, three-slit interference, four-slit interference, etc. (Duarte, 1991, 2002, 2005). For reviews see Tunable Laser Optics and Tunable Laser Applications).

The 1991 and 1993 papers also reported, for the first time, on the use of quantum mechanics techniques, via Dirac's notation, in the field of imaging. In addition, these papers illustrated the prediction of measured interferograms using interferometric equations derived using Dirac's quantum notation.

It should be noted that this class of very thin one-dimensional illumination has also become known in the community as light sheet illumination and selective plane illumination and is mainly accomplished via the use of cylindrical lenses. However, the use of cylindrical lenses does not provide the extreme expansion ratios achieved via the use of multiple-prism beam exansion.

• F. J. Duarte and I. E. Olivares, N-slit quantum interferometers in the nanometer domain, Appl. Phys. B 129, 88 (2023).
  
  
• F. J. Duarte, Fundamentals of Quantum Entanglement, 2nd Edn(Institute of Physics, Bristol, 2022).
  
  

• F. J. Duarte, Fundamentals of Quantum Entanglement (Institute of Physics, Bristol, 2019).
  
  

• F. J. Duarte, Organic molecules in photonics, cancer phototherapy, and neurophotonics, in Neurophotonics and Biomedical Spectroscopy R. R. Alfano and L. Shi (Eds.) (Elsevier, New York, 2019).
• F. J. Duarte, Interferometric imaging, in Tunable Laser Applications, Third Edition, F. J. Duarte (Ed.) (CRC, New York, 2016) Chapter 10.
  
  
• F. J. Duarte, Tunable laser microscopy, in Tunable Laser Applications, Third Edition, F. J. Duarte (Ed.) (CRC, New York, 2016) Chapter 9.
  
  

• F. J. Duarte and T. S. Taylor, Laser Focus World, Quantum entanglement physics secures space-to-space interferometric communications, 51(4), 54-58 (2015).

• F. J. Duarte, Tunable Laser Optics, 2nd Edition (CRC, New York, 2015).

• F. J. Duarte, Quantum Optics for Engineers (CRC, New York, 2014).

• F. J. Duarte, Tunable laser optics: applications to optics and quantum optics, Progress in Quantum Electronics 37, 326-347 (2013) (Invited).
  
  
• F. J. Duarte, T.S. Taylor, A.M. Black, I.E. Olivares, Diffractive patterns superimposed over propagating N-slit interferograms, J. Mod. Opt. 60, 136-140 (2013).

• F. J. Duarte, T. S. Taylor, A. M. Black, W. E. Davenport, and P. G. Varmette, N-slit interferometer for secure free-space optical communications: 527 m intra interferometric path length , J. Opt. 13, 035710 (2011).
  
  
• F. J. Duarte, Triple-slit experiment, Optics & Photonics News 22(12), 7 (2010).
  
  
• F. J. Duarte, T. S. Taylor, A. B. Clark, and W. E. Davenport, The N-slit interferometer: an extended configuration, J. Opt. 12, 015705 (2010).
  
  
• F. J. Duarte, K. M. Vaeth, and L. S. Liao, Electrically excited organic light-emitting diodes with spatial and spectral coherence, US Patent 7667391 (February 23, 2010).
  
  
• F. J. Duarte, Interferometric imaging, in Tunable Laser Applications, 2nd Ed., F. J. Duarte (Ed.) (CRC, New York, 2009) Chapter 12.
  
  
• F. J. Duarte, Secure interferometric communications in free space: enhanced sensitivity for propagation in the metre range, J. Opt. A: Pure Appl. Opt. 7, 73-75 (2005).
  
  
• F. J. Duarte, Comment on "Reflection, refraction, and multislit interference," Eur. J. Phys. 25, L57-L58 (2004).^*****
  
  
• F. J. Duarte, Tunable Laser Optics (Elsevier-Academic, New York, 2003) Chapter 2.
  
  
• F. J. Duarte, Secure interferometric communications in free space, Opt. Commun. 205, 313-319 (2002).^**
  
  
• F. J. Duarte, Laser sensitometer using multiple-prism beam expansion and a polarizer, US Patent 6236461 (22nd of May, 2001).
  
  
• F. J. Duarte, Answer to question #60. Interference of two independent sources,Am. J. Phys. 66, 662-663 (1998).^***
  
  
• F. J. Duarte, Interference, diffraction, and refraction, via Dirac's notation, Am. J. Phys. 65, 637-640 (1997).
  
  
• F. J. Duarte, Generalized interference and optical processing, in Proceedings of the International Conference on Lasers '95, V. J. Corcoran and T. A. Goldman (Eds.) (STS, McLean, Va, 1996) pp. 615-617.
  
  
• F. J. Duarte, Interferometric imaging, in Tunable Laser Applications, F. J. Duarte (Ed.) (Marcel-Dekker, New York, 1995) Chapter 5.
  
  
• F. J. Duarte, On a generalized interference equation and interferometric measurements, Opt. Commun. 103, 8-14 (1993).^**
  
  
• F. J. Duarte, Electro-optical interferometric microdensitometer system, US Patent 5255069 (19th of October, 1993)..^**
  
  
• F. J. Duarte, Cavity dispersion equation Δλ ≈ Δθ(∂θ/∂λ) ^{– 1}: a note on its origin, Appl. Opt. 31, 6979-6982 (1992).^*
  
  
• F. J. Duarte, Dispersive dye lasers, in High Power Dye Lasers, F. J. Duarte (Ed.) (Springer-Verlag, Berlin, 1991) pp. 7-43.
  
  
• F. J. Duarte and D. J. Paine, Quantum mechanical description of N-slit interference phenomena, in Proceedings of the International Conference on Lasers '88, R. C. Sze and F. J. Duarte (Eds.) (STS, McLean, Va, 1989) pp. 42-47.
  
  
• F. J. Duarte, Beam shaping with telescopes and multiple-prism beam expanders, J. Opt. Soc. Am. A 4, P30 (1987).

Keywords: double-slit interference, double-slit interferometer, double-slit interferometry, four-slit interference, four-slit interferometer, four-slit interferometry, N-slit interference, N-slit interferometer, N-slit interferometry, quantum, quantum imaging, two-slit interference, two-slit interferometer, two-slit interferometry, three-slit interference, three-slit interferometer, three-slit interferometry, triple-slit interference, triple-slit interferometer, triple-slit interferometry, quantum interference